Correlation
When the two sets of data are strongly linked together we say they have a High Correlation.
The word Correlation is made of Co- (meaning "together"), and Relation
- Correlation is Positive when the values increase together, and
- Correlation is Negative when one value decreases as the other increases
Like this:
(Learn More About Correlation)
Negative Correlation
Correlations can be negative, which means there is a correlation but one value goes down as the other value increases.
Example : Birth Rate vs IncomeThe birth rate tends to be lower in richer countries.Below is a scatter plot for about 100 different countries. |
|
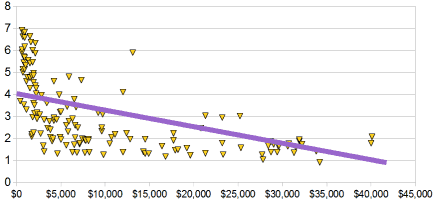
It has a negative correlation (the line slopes down)
https://www.mathsisfun.com/data/scatter-xy-plots.html
ALGEBRA 2
Rationals
BASICS: (top)
SIMPLIFYING: (top)
-> see also: Factoring & Expanding
Simplifying rational expressions is fundamental to graphing rational functions and solving rational equations. To simplify a rational expression, follow these steps:
- Factor the polynomials in both the numerator and denominator
- Cancel out like terms
- Keep track of restrictions on domain
This last step is extremely important. If a certain value of x makes the denominator of the rational 0 at any point, the rational is undefined at that point and it is not part of the domain. Let's consider an example.
EXAMPLE 1:Problem: Simplify the rational function.
Solution: First, factor the numerator and denominator to get. Then, cancel out the common term (x+2) to get the simplified function
. This is not the full answer, however; the domain of this function is restricted. If one plugs either the point x=2 or x=-2 to this simplified function, one finds f(0)=0. This is not correct, though, as if either x=2 or x=-2 is plugged into the original function one finds
, which is undefined. Thus, one simplifying, one must include the domain restrictions. The final answer, then, is
.
EXAMPLE 2:Problem: Simplify the rational function.
Solution: First, rewrite the division as multiplication and factor everything:. Then, cancel out identical terms, keeping track of the restrictions from the denominator (x≠5, -2, -3) to get
.
GRAPHING: (top)
-> see also: Manipulating Graphs
Graphing rationals has much in common with graphing polynomials. As with polynomials, we wish to show end behavior, the y-intercept, x-intercepts, and behavior at roots. The y-intercept, x-intercepts, and behavior at roots are found the same way for rational functions as for polynomials. There are differences in end behavior, and we are also concerned with asymptotes, which are not found in graphs of polynomials.
End Behavior
The end behavior of rational functions is more complicated than that of polynomials and is easiest to study if broken down into cases.
Case 1: Degree in Denominator is Greater than Degree in Numerator
In this case, where the degree of the denominator is greater than the degree in the numerator, 
. As 
, the polynomial in the denominator grows much faster than the polynomial in the numerator, so their quotient approaches to 0.
. As , the polynomial in the denominator grows much faster than the polynomial in the numerator, so their quotient approaches to 0.
The image below shows a graph of 
showing this end behavior.
showing this end behavior.
Case 2: Same Degree in Numerator and Denominator
As , only the first term (term with the greatest degree) in each polynomial becomes significant. That is, for a rational function such as 
, we can determine the end behavior by looking at the function 
. Since 
, 
and 
. This results in a horizontal asymptote at y=1 that the graph approaches but never reaches as .
, only the first term (term with the greatest degree) in each polynomial becomes significant. That is, for a rational function such as , we can determine the end behavior by looking at the function . Since , and . This results in a horizontal asymptote at y=1 that the graph approaches but never reaches as .
To sum up, the end behavior of a rational function with the same degree in the numerator and denominator is determined by the quotient of the leading coefficients of the two polynomials.
EXAMPLE 3:Problem: State the end behavior of the rational.
Solution: First, for visual clarity, rearrange the terms so that the leading term of each polynomial is written first:. As
. The x3 terms cancel out, leaving
. Thus,
. The image below is a graph of
exhibiting this end behavior.
Note that the graph can cross the asymptote, which it does in this example. It then approaches the asymptote from the opposite side as
Case 3: Degree in Numerator is Greater than Degree In Denominator
There is one special case when the degree in the numerator of a rational is greater than the degree in the denominator that deserves our immediate attention. In this special case, the degree of the numerator is only greater than the degree of the denominator by one and a common factor cancels out, such has in the rational 
. Here, the (x-3) term cancels out, giving 
. Thus, the graph is identical to that of the linear function 
, except that the graph of f(x) has a point discontinuity at x=3. In this special case, then, the end behavior of the function is identical to that of a linear function; namely, 
and 
. This is shown in the graph of 
below.
. Here, the (x-3) term cancels out, giving . Thus, the graph is identical to that of the linear function , except that the graph of f(x) has a point discontinuity at x=3. In this special case, then, the end behavior of the function is identical to that of a linear function; namely, and . This is shown in the graph of below.
In most situations where the degree in the numerator is greater than the degree in the denominator, there are not terms that can be cancelled out. To graph these rationals, one must determine their end behavior using polynomial long division or synthetic division. This division will result in an expression of the form 
where R(x) is the remainder after the division (and Q(x) is the polynomial in the denominator). Because the degree of Q(x) is greater than the degree of R(x), 
. Since 
and , 
. This resulting linear function y=ax+b is called an oblique asymptote. Note that the limit of oblique asymptote, and thus of the original rational, is 
and 
if a>0 and the opposite if a<0, so it is correct to say that and (for a >0; or the opposite for a<0). It is more specific and useful, particularly for graphing, however, to find the oblique asymptote. This procedure will become more clear with an example.
where R(x) is the remainder after the division (and Q(x) is the polynomial in the denominator). Because the degree of Q(x) is greater than the degree of R(x), . Since and , . This resulting linear function y=ax+b is called an oblique asymptote. Note that the limit of oblique asymptote, and thus of the original rational, is and if a>0 and the opposite if a<0, so it is correct to say that and (for a >0; or the opposite for a<0). It is more specific and useful, particularly for graphing, however, to find the oblique asymptote. This procedure will become more clear with an example.EXAMPLE 4:Problem: Find the oblique asymptote of the rational.
Solution: First note that there are no common factors in the numerator and denominator that can be cancelled out. Next, use long division or synthetic division to find. Thus, there is an oblique asymptote at y=x+2. This is shown in the graph below.
Vertical Asymptotes
You probably noticed a number of vertical lines in the graphs of rationals in the preceding examples. These are vertical asymptotes, which result when the denominator of a rational that has no common factors to cancel in its numerator and denominator is equal to zero. The function is undefined at this point.
How do graphs behave around vertical asymptotes? There are two possibilities. As x approaches the asymptote, the graph approaches either positive or negative infinity, as shown in the image at right. The notation 
and 
are used to denote the behavior of a graph as it approaches an asymptote at x=C from the left and right respectively. Thus, for the graph at right, 
and 
.
Or course, we wish to be able to predict how a graph will behave around a particular vertical asymptote. As with roots, the behavior around the asymptote is determined by its multiplicity. If the multiplicity of the asymptote is odd, the behavior across the asymptote is opposite; if the graph approaches positive infinity from one side, it must approach negative infinity from the other. If the multiplicity of the asymptote is even, however, the graph has the same limit regardless of which time direction it approaches the asymptote. These two possibilities are shown below.
Note that you only know that the behavior will be the same or opposite; additional information, such as the y-intercept and roots, must be used to determine where the graph goes up and down.
Determining the end behavior, y-intercept, x-intercepts, behavior at roots, and vertical asymptotes of a rational function can yield a useful graph of the function. Putting all of this information together to develop a coherent picture of what's going on can take some time; the best way to get better at it is to practice with problems.
https://www.math.brown.edu/utra/rationals.html
No comments:
Post a Comment