Week of October 2, 2017

Foundations of Algebra

Part to Part and Part to Whole Ratios

Take a look at the following problems.

Data Sufficiency: What was Company X’s percentage profit in 2011?

1) The ratio of costs to profits for Company X was 3 to 1 in 2011.

2) Company X’s costs in 2011 were $360,000.

A recipe for punch calls for 4 parts seltzer to one part juice. If John wants to make 5 gallons of punch, how many 8 ounce cans of juice does he need (1 gallon = 128 ounces)?

A) 32

B) 20

C) 16

D) 10

E) 8

Both these problems have something in common. We are given a ratio, but not the specific ratio that would be most useful to our calculation. In the first case, the percentage profit can be calculated at profit/revenue * 100 (remember revenue = profit + costs). In the second case, the ratio that would be most helpful to solving is the ratio of juice to punch, which we could then use to calculate how many ounces of juice we need. It is common for test takers to get stuck at this point not knowing how to get to the ratio needed, but with a little understanding of ratios, it is actually a rather simple task to convert from the given ratios to the ratios that would be most helpful.

Ratios can be divided into part-to-part ratios and part-to-whole ratios. Part-to-part ratios provide the relationship between two distinct groups. For example, the ratio of men to women is 3 to 5, or the solution contains 3 parts water for every 2 parts alcohol.

Part-to-whole ratios provide the relationship between a particular group and the whole populations (including the particular group). For example, 3/5 of the students in the class are girls, or the mixture is 40% rye grass (40% is equivalent to saying 40 of every 100 parts).

If the population for a given problem consists of only two parts, A and B, there are three ratios you can potentially write, one part-to-part ratio and two part-to-whole ratios. Keep in mind that since there are only two parts, the whole is just the sum of these two parts, or A + B:

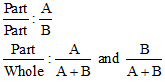
You could write the ratio of B/A, but that is just the reciprocal of the part-to-part ratio already written. In this universe with only two parts, given any one of the three above ratios, you can calculate the other two.

For example, if the ratio of men to women is 3 to 5, we know A=3 and B=5. From here, we can calculate all three ratios (3/5, 3/8, and 5/8). You can also calculate all three ratios if given only one of the part-to-whole ratios. The mixture of flour and sugar is made up of 5/7 flour. In this case, A = 5, A+B=7 making B = 5 -7 = 2.

Recognizing that you can get any of these three ratios given any one is valuable in solving problems because in particular cases, one of the ratios may be more useful to your calculation. Keep in mind that the rules above only apply in cases where there are only two parts. The calculations are not possible in ratios with three or more parts. For example, if a pet store has dogs, cats, and birds, and you are given that 3/7 of the animals are dogs—you cannot calculate the part-to-part ratio of dogs to cats, for example (note: you can calculate the ratio of dogs to cats and birds collectively is 3 to 4).

Let’s take this new knowledge back to our problems from the beginning of this post. In the first problem, statement 1 provides the part-to-part ratio (A = costs, B = profits, A + B = revenues). We are looking for the part-to-whole ratio of B to A+B, so clearly this statement is sufficient. Statement 2 is not sufficient, meaning the answer is A.

For problem 2, we need to calculate how much juice is needed. The total volume of liquid is 640 ounces (5 gallons * 128 ounces per gallon). To calculate the amount of juice, we need the part-to-whole ratio of juice to punch. We are given the part-to-part ratio of seltzer to juice as 4 to 1. This ratio and the formulas provided above enable us to calculate the part-to-whole ratio of juice to punch as 1 to 5. Multiplying this ratio by the total volume of liquid shows that 128 ounces of juice are needed. The final step in solving is dividing 128 by the 8 ounces per can, giving answer C) 16.

This knowledge of ratios is also helpful in working with probability problems. Remember that the definition of probability is successes/total outcomes, a specific part-to-whole ratio. There are only two parts in the case of probability, successes and failures. As such, if you can count the successes and count the failures, you can convert to the correct part-to-whole ratio to calculate the probability:

https://www.manhattanprep.com/gmat/blog/2012/08/01/part-to-part-and-part-to-whole-ratios/

Anatomy

The CNS and PNS

     The nervous system has two different major parts. The two parts are the central nervous system and the peripheral nervous. The central nervous system or the CNS contains the brain and the spinal cord. All together, the brain and the spinal cord serve the nervous system's command station. When the sensory input reaches the CNS, the spinal cord and the brain figure outs what it exactly means. After, they quickly orders out the body parts that needs to move faster.
     Everything else but the CNS it is known as the peripheral nervous system. The peripheral nervous system or PNS contains the nerves, which leave the brain and the spinal cord and travel to certain areas of the body. The peripheral nervous system's main job is to send information gathered by the body's sensory receptors to the CNS as quickly as possible. Once the CNS has understood the information, the PNS will relay the specific orders back out the body. These nerves which carry information in a way of nerve impulses to and from the brain are called cranial nerves. The nerves that carry impulse to and are carrying information from the spine are called spinal nerves. 
     The PNS has two important parts. They are the motor division and the sensory division. The sensory division collects the impulses from the sensory receptors in areas like skin, muscles, and organs, and also carries those impulses through the nerves to the CNS. The motor division collects the outgoing messages from the CNS and delivers them to the appropriate body organs, telling them what to do. The motor division does the opposite from the sensory division. 

http://www.gwinnett.k12.ga.us/LilburnES/PromoteGA/biochemistry/Brain_CNS.html

Geometry

Circles and Volume Part One Key Terms

Arc - an unbroken part of a circle

Arc measure - the measure of the angle at the center of the circle that creates an arc

Major arc - an arc whose measure is greater than 180 degrees

Minor arc - an arc whose measure is less than 180 degrees

Point of tangency - the point where a tangent line touches the circle

Chord - a segment whose endpoints both lie on the circle

Central angle - an angle whose vertex is at the center of the circle

Common tangent - a line that is tangent to two coplanar circles

Cyclic quadrilaterals - a quadrilateral inscribed in a circle (all 4 vertices lie on the circle)

Inscribed angle - an angle whose vertex is on the circle and whose sides are made up by chords of a circle

Circumscribed angle - an angle whose rays are tangent to a circle

Circumscribed circle - a circle containing an inscribed polygon

Inscribed polygon - a polygon contained inside a circle whose vertices all lie on the circle

Inscribed circle - a circle contained in a polygon where each side of the polygon is tangent to the circle

Secant line - a line in the plane of the circle that intersects it at exactly two points

Semicircle - a half circle; created by an arc whose measure is equal to 180 degrees

Tangent line - a line in the plane of the circle that intersects it at exactly one point

Circumcenter - the point of intersection of the perpendicular bisectors of a triangle; this is also the center of the circle circumscribed around a given triangle