Week of February 12, 2018

Algebra 2

Composition of Functions

"Function Composition" is applying one function to the results of another:

The result of f() is sent through g()

It is written: (g º f)(x)

Which means: g(f(x))

Example: f(x) = 2x+3 and g(x) = x²

"x" is just a placeholder. To avoid confusion let's just call it "input":

f(input) = 2(input)+3

g(input) = (input)²

Let's start:

(g º f)(x) = g(f(x))

First we apply f, then apply g to that result:

(g º f)(x) = (2x+3)²

What if we reverse the order of f and g?

(f º g)(x) = f(g(x))

First we apply g, then apply f to that result:

(f º g)(x) = 2x²+3

We get a different result!
So be careful which function comes first.

Symbol

The symbol for composition is a small circle:

(g º f)(x)

It is not a filled in dot: (g · f)(x), as that means multiply.

Composed With Itself

We can even compose a function with itself!

Example: f(x) = 2x+3

(f º f)(x) = f(f(x))

First we apply f, then apply f to that result:

(f º f)(x) = 2(2x+3)+3 = 4x + 9

We should be able to do it without the pretty diagram:

(f º f)(x)= f(f(x))

 = f(2x+3)

 = 2(2x+3)+3

 = 4x + 9

Domains

It has been easy so far, but now we must consider the Domains of the functions.

The domain is the set of all the values that go into a function.

The function must work for all values we give it, so it is up to us to make sure we get the domain correct!

Example: the domain for √x (the square root of x)

We can't have the square root of a negative number (unless we use imaginary numbers, but we aren't), so we must exclude negative numbers:

The Domain of √x is all non-negative Real Numbers

On the Number Line it looks like:

Using set-builder notation it is written:

{ x | x ≥ 0}

Or using interval notation it is:

[0,+∞)

It is important to get the Domain right, or we will get bad results!

Domain of Composite Function

We must get both Domains right (the composed function and the first function used).

When doing, for example, (g º f)(x) = g(f(x)):

• Make sure we get the Domain for f(x) right,
• Then also make sure that g(x) gets the correct Domain

Example: f(x) = √x and g(x) = x²

The Domain of f(x) = √x is all non-negative Real Numbers

The Domain of g(x) = x² is all the Real Numbers

The composed function is:

(g º f)(x)= g(f(x))

 = (√x)²

 = x

Now, "x" normally has the Domain of all Real Numbers ...

... but because it is a composed function we must also consider f(x),

So the Domain is all non-negative Real Numbers

Why Both Domains?

Well, imagine the functions are machines ... the first one melts a hole with a flame (only for metal), the second one drills the hole a little bigger (works on wood or metal):

What we see at the end is a drilled hole, and we may think "that should work for wood or metal". But if we put wood into g º f then the first function f will make a fire and burn everything down!

So what happens "inside the machine" is important.

De-Composing Function

We can go the other way and break up a function into a composition of other functions.

Example: (x+1/x)²

That function can be made from these two functions:

f(x) = x + 1/x

g(x) = x²

And we get:

(g º f)(x)= g(f(x))

 = g(x + 1/x)

 = (x + 1/x)²

This can be useful if the original function is too complicated to work on.

Summary

"Function Composition" is applying one function to the results of another.
(g º f)(x) = g(f(x)), first apply f(), then apply g()
We must also respect the domain of the first function
Some functions can be de-composed into two (or more) simpler functions.

 https://www.mathsisfun.com/sets/functions-composition.html

Algebra 1

Elimination Method

The elimination method of solving systems of equations is also called the addition method. To solve a system of equations by elimination we transform the system such that one variable "cancels out".

Example 1: Solve the system of equations by elimination

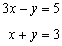
Solution:

In this example we will "cancel out" the y term. To do so, we can add the equations together.

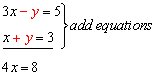
Now we can find: x = 2

In order to solve for y, take the value for x and substitute it back into either one of the original equations.

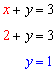
The solution is (x, y) = (2, 1).

Example 2: Solve the system using elimination

Solution:

Look at the x - coefficients. Multiply the first equation by -4, to set up the x-coefficients to cancel.

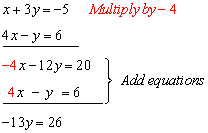
Now we can find: y = -2

Take the value for y and substitute it back into either one of the original equations.

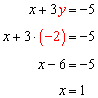
The solution is (x, y) = (1, -2).

Example 3: Solve the system using elimination method

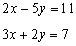
Solution:

In this example, we will multiply the first row by -3 and the second row by 2; then we will add down as before.

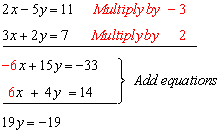
Now we can find: y = -1

Substitute y = -1 back into first equation:

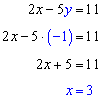
The solution is (x, y) = (3, -1).

https://www.mathportal.org/algebra/solving-system-of-linear-equations/elimination-method.php