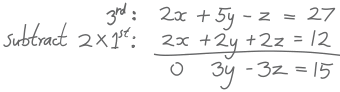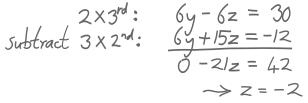Systems of Linear Equations
A linear equation is not always in the form y = 3.5 − 0.5x,
It can also be like y = 0.5(7 − x)
Or like y + 0.5x = 3.5
Or like y + 0.5x − 3.5 = 0 and more.
(Note: those are all the same linear equation!)
A System of Linear Equations is when we have two or more linear equations working together.
A linear equation is not always in the form y = 3.5 − 0.5x,
It can also be like y = 0.5(7 − x)
Or like y + 0.5x = 3.5
Or like y + 0.5x − 3.5 = 0 and more.
(Note: those are all the same linear equation!)
A System of Linear Equations is when we have two or more linear equations working together.
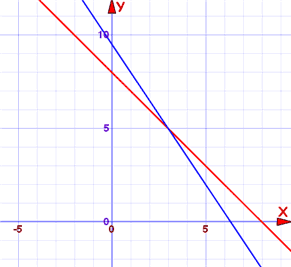
Example: Here are two linear equations:
Let's try to build and solve a real world example:
Let's try to build and solve a real world example:
Example: You versus Horse
So now you know what a System of Linear Equations is.
Let us continue to find out more about them ....
So now you know what a System of Linear Equations is.
Let us continue to find out more about them ....
Solving
There can be many ways to solve linear equations!
Let us see another example:
There can be many ways to solve linear equations!
Let us see another example:
Example: Solve these two equations:
Linear Equations
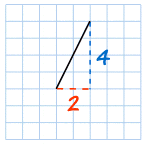
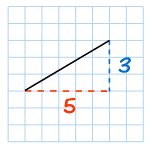
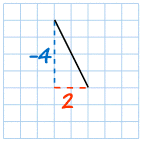
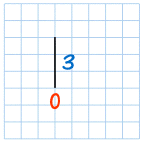
Only simple variables are allowed in linear equations. No x2, y3, √x, etc:

Linear vs non-linear
Only simple variables are allowed in linear equations. No x2, y3, √x, etc:

Linear vs non-linear
Dimensions
A Linear Equation can be in 2 dimensions ...
(such as x and y) 
... or in 3 dimensions ...
(it makes a plane) 
... or 4 dimensions ... ... or more!
| A Linear Equation can be in 2 dimensions ... (such as x and y) |  | |
| ... or in 3 dimensions ... (it makes a plane) |  | |
| ... or 4 dimensions ... | ||
| ... or more! |
Common Variables
For the equations to "work together" they share one or more variables:
A System of Equations has two or more equations in one or more variables
For the equations to "work together" they share one or more variables:
A System of Equations has two or more equations in one or more variables
Many Variables
So a System of Equations could have many equations and many variables.
So a System of Equations could have many equations and many variables.
Example: 3 equations in 3 variables
There can be any combination:
- 2 equations in 3 variables,
- 6 equations in 4 variables,
- 9,000 equations in 567 variables,
- etc.
- 2 equations in 3 variables,
- 6 equations in 4 variables,
- 9,000 equations in 567 variables,
- etc.
There can be any combination:
Solutions
When the number of equations is the same as the number of variables there is likely to be a solution. Not guaranteed, but likely.
In fact there are only three possible cases:
- No solution
- One solution
- Infinitely many solutions
When there is no solution the equations are called "inconsistent".
One or infinitely many solutions are called "consistent"
Here is a diagram for 2 equations in 2 variables:

- No solution
- One solution
- Infinitely many solutions
When the number of equations is the same as the number of variables there is likely to be a solution. Not guaranteed, but likely.
In fact there are only three possible cases:
When there is no solution the equations are called "inconsistent".
One or infinitely many solutions are called "consistent"
Here is a diagram for 2 equations in 2 variables:

Independent
"Independent" means that each equation gives new information.
Otherwise they are "Dependent".
Also called "Linear Independence" and "Linear Dependence"
"Independent" means that each equation gives new information.
Otherwise they are "Dependent".
Otherwise they are "Dependent".
Also called "Linear Independence" and "Linear Dependence"
Example:
Where the Equations are True
The trick is to find where all equations are true at the same time.
True? What does that mean?
The trick is to find where all equations are true at the same time.
True? What does that mean?
Example: You versus Horse
So they have to be true simultaneously ...
... that is why some people call them "Simultaneous Linear Equations"
So they have to be true simultaneously ...
... that is why some people call them "Simultaneous Linear Equations"
Solve Using Algebra
It is common to use Algebra to solve them.
Here is the "Horse" example solved using Algebra:
It is common to use Algebra to solve them.
Here is the "Horse" example solved using Algebra:
Example: You versus Horse
Algebra vs Graphs
Why use Algebra when graphs are so easy? Because:
More than 2 variables can't be solved by a simple graph.
So Algebra comes to the rescue with two popular methods:
- Solving By Substitution
- Solving By Elimination
We will see each one, with examples in 2 variables, and in 3 variables. Here goes ...
- Solving By Substitution
- Solving By Elimination
Why use Algebra when graphs are so easy? Because:
More than 2 variables can't be solved by a simple graph.
So Algebra comes to the rescue with two popular methods:
We will see each one, with examples in 2 variables, and in 3 variables. Here goes ...
Solving By Substitution
These are the steps:
- Write one of the equations so it is in the style "variable = ..."
- Replace (i.e. substitute) that variable in the other equation(s).
- Solve the other equation(s)
- (Repeat as necessary)
Here is an example with 2 equations in 2 variables:
- Write one of the equations so it is in the style "variable = ..."
- Replace (i.e. substitute) that variable in the other equation(s).
- Solve the other equation(s)
- (Repeat as necessary)
These are the steps:
Here is an example with 2 equations in 2 variables:
Example:
Solving By Substitution: 3 equations in 3 variables
OK! Let's move to a longer example: 3 equations in 3 variables.
This is not hard to do... it just takes a long time!
OK! Let's move to a longer example: 3 equations in 3 variables.
This is not hard to do... it just takes a long time!
Example:
We can use this method for 4 or more equations and variables... just do the same steps again and again until it is solved.
Conclusion: Substitution works nicely, but does take a long time to do.
We can use this method for 4 or more equations and variables... just do the same steps again and again until it is solved.
Conclusion: Substitution works nicely, but does take a long time to do.
Solving By Elimination
Elimination can be faster ... but needs to be kept neat.
"Eliminate" means to remove: this method works by removing variables until there is just one left.
The idea is that we can safely:
- multiply an equation by a constant (except zero),
- add (or subtract) an equation on to another equation
Like in these examples:

- multiply an equation by a constant (except zero),
- add (or subtract) an equation on to another equation
Elimination can be faster ... but needs to be kept neat.
"Eliminate" means to remove: this method works by removing variables until there is just one left.
The idea is that we can safely:
Like in these examples:

WHY can we add equations to each other?
Imagine two really simple equations:
x − 5 = 3
5 = 5
We can add the "5 = 5" to "x − 5 = 3":
x − 5 + 5 = 3 + 5
x = 8
Try that yourself but use 5 = 3+2 as the 2nd equation
It will still work just fine, because both sides are equal (that is what the = is for!)
We can also swap equations around, so the 1st could become the 2nd, etc, if that helps.
OK, time for a full example. Let's use the 2 equations in 2 variables example from before:
Imagine two really simple equations:
It will still work just fine, because both sides are equal (that is what the = is for!)
x − 5 = 3
5 = 5
We can add the "5 = 5" to "x − 5 = 3":5 = 5
x − 5 + 5 = 3 + 5
x = 8
Try that yourself but use 5 = 3+2 as the 2nd equationx = 8
It will still work just fine, because both sides are equal (that is what the = is for!)
We can also swap equations around, so the 1st could become the 2nd, etc, if that helps.
OK, time for a full example. Let's use the 2 equations in 2 variables example from before:
Example:
Here is another example:
Here is another example:
Example:
And lastly:
And lastly:
Example:
And so now we have seen an example of each of the three possible cases:
- No solution
- One solution
- Infinitely many solutions
- No solution
- One solution
- Infinitely many solutions
And so now we have seen an example of each of the three possible cases:
Solving By Elimination: 3 equations in 3 variables
Before we start on the next example, let's look at an improved way to do things.
Follow this method and we are less likely to make a mistake.
First of all, eliminate the variables in order:
- Eliminate xs first (from equation 2 and 3, in order)
- then eliminate y (from equation 3)
So this is how we eliminate them:
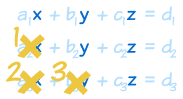 We then have this "triangle shape":
We then have this "triangle shape":
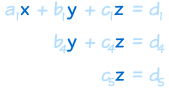 Now start at the bottom and work back up (called "Back-Substitution")
Now start at the bottom and work back up (called "Back-Substitution")
(put in z to find y, then z and y to find x):
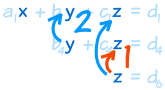 And we are solved:
And we are solved:
 ALSO, we will find it is easier to do some of the calculations in our head, or on scratch paper, rather than always working within the set of equations:
ALSO, we will find it is easier to do some of the calculations in our head, or on scratch paper, rather than always working within the set of equations:
- Eliminate xs first (from equation 2 and 3, in order)
- then eliminate y (from equation 3)
Before we start on the next example, let's look at an improved way to do things.
Follow this method and we are less likely to make a mistake.
First of all, eliminate the variables in order:
So this is how we eliminate them:
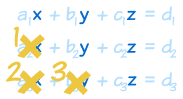
We then have this "triangle shape":
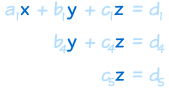
Now start at the bottom and work back up (called "Back-Substitution")
(put in z to find y, then z and y to find x):
(put in z to find y, then z and y to find x):
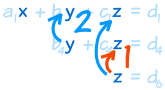
And we are solved:

ALSO, we will find it is easier to do some of the calculations in our head, or on scratch paper, rather than always working within the set of equations:
Example:
General Advice
Once you get used to the Elimination Method it becomes easier than Substitution, because you just follow the steps and the answers appear.
But sometimes Substitution can give a quicker result.
- Substitution is often easier for small cases (like 2 equations, or sometimes 3 equations)
- Elimination is easier for larger cases
And it always pays to look over the equations first, to see if there is an easy shortcut ... so experience helps.
- Substitution is often easier for small cases (like 2 equations, or sometimes 3 equations)
- Elimination is easier for larger cases
Once you get used to the Elimination Method it becomes easier than Substitution, because you just follow the steps and the answers appear.
But sometimes Substitution can give a quicker result.
And it always pays to look over the equations first, to see if there is an easy shortcut ... so experience helps.